Lecture 3 Discussion (Coordinate Systems & Vectors & Measurement & Units)

[1] The position of a particle moving under uniform acceleration is some function of time and the acceleration. Suppose we write this position s = ka^m tⁿ where , k is a dimensionless constant. Show by dimensional analysis that this expression is satisfied if if m = 1 and n = 2

[2] Newton’s law of universal gravitation is represented by

Here F is the magnitude of the gravitational force exerted by one small object on another , M and m are the masses of the objects, and r is a distance. Force has the SI units kg ·m/ s2. What are the SI units of the proportionality constant G?

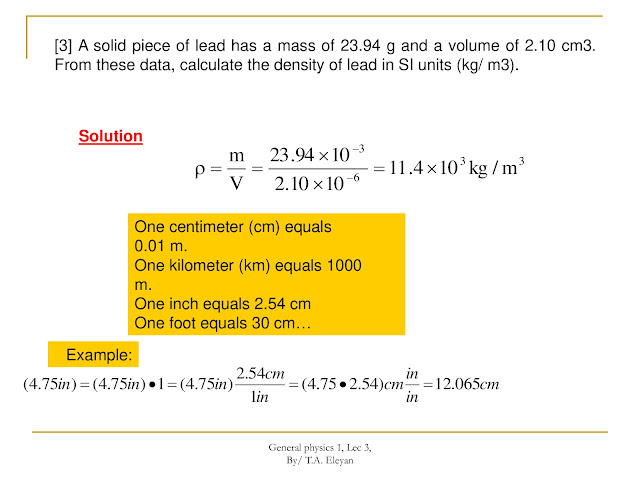
[3] A solid piece of lead has a mass of 23.94 g and a volume of 2.10 cm3. From these data, calculate the density of lead in SI units (kg/ m3).

[4] If the rectangular coordinates of a point are given by (2, y) and its polar coordinates are ( r , 30°), determine y and r.

[5] Vector A has a magnitude of 8.00 units and makes an angle of 45.0 ° with the positive x axis. Vector B also has a magnitude of 8.00 units and is directed along the negative x axis. Using graphical methods, find (a) the vector sum A + B and (b) the vector difference A - B.

[6] Given the vectors A = 2.00 i +6.00 j and B = 3.00 i - 2.00 j, (a) draw the vector sum , C = A + B and the vector difference D = A - B. (b) Calculate C and D, first in terms of unit vectors and then in terms of polar coordinates, with angles measured with respect to the , +x axis.

[7] Consider the two vectors A = 3 i - 2 j and B = i - 4 j. Calculate (a) A + B, (b) A - B, (c) │A + B│, (d) │A - B│, and (e) the directions of A + B and A - B.

[8] The vector A has x, y, and , z components of 8.00, 12.0, and -4.00 units, respectively. (a) Write a vector expression for A in unit vector notation. (b) Obtain a unit vector expression for a vector B four time the length of A pointing in the same direction as A. (c) Obtain a unit vector expression for a vector C three times the length of A pointing in the direction opposite the direction of A.

[9] Three displacement vectors of a croquet ball are shown in Figure, where A = 20.0 units, B = 40.0 units, and C = 30.0 units. Find the magnitude and direction of the resultant displacement

[10] Find the magnitude and the direction of resultant force